Fragile crystals could help create better, tunable electronics
Experimental and theoretical physics meet again.
Coffee cups and donuts are one and the same, according to mathematicians. While they might have some serious physical differences (crunching on a coffee cup would be significantly less pleasant than biting into a donut,) their underlying topology, or the way their surfaces bend, are the same because they both have only one hole. These similarities may seem semantic, but making use of topology has enabled scientists to explore futuristic materials and their uses in high-performance technologies and quantum computing. But, a golden-child of topological technology, a material called a topological insulator, might be more mysterious than physicists first believed.
In recent years scientists have found that this material, which is prized for its ability to conduct electron flow on its surface while insulating flow from its center, may actually be more fragile than it seems and that its topological barriers may in fact be more free-flowing and less predictable. But, far from letting that news discourage them, scientists in a pair of new studies have worked to uncover how such fragile topology may actually be useful.
The first study, published Thursday in the journal Science, used mathematical modeling in order to better understand this material's fragile properties. The team focused on the quantum behavior of electrons both on the surface and in the interior of the topological insulator and how it might be possible in some cases for this strict topological barrier to breakdown. Typically in these materials, the wave function (think an electron GPS signal) of electrons in the center of the insulator spread neatly to the edge of the surface, creating a boundary-correspondence that allows for free flow on the surface and insulation in the center. But, by looking at these electrons more closely, the team discovered that this wasn't always the case. The researchers call this scenario a "twisted bulk-boundary-correspondence" and it creates a fragile topology in which electrons cannot flow on the surface of the material.
B. Andrei Bernevig, a professor of physics at Princeton and co-author on both papers, said in a statement that this phenomenon is a breakdown of how researchers typically believe these materials behave.
"Fragile topology is a strange beast: It is now predicted to exist in hundreds of materials," said Bernevig. "It is as if the usual principle that we have been relying on to experimentally determine a topological state breaks down."
In addition to identifying mechanisms that might be causing these strange fragile topologies, the authors also identified that these wave functions could actually be manipulated to change the boundary-correspondence conditions of the material. The researchers say that this ability to tune the material, essentially turning on and off the conductivity of its surface, could be a useful design element for electronic and optical technologies.
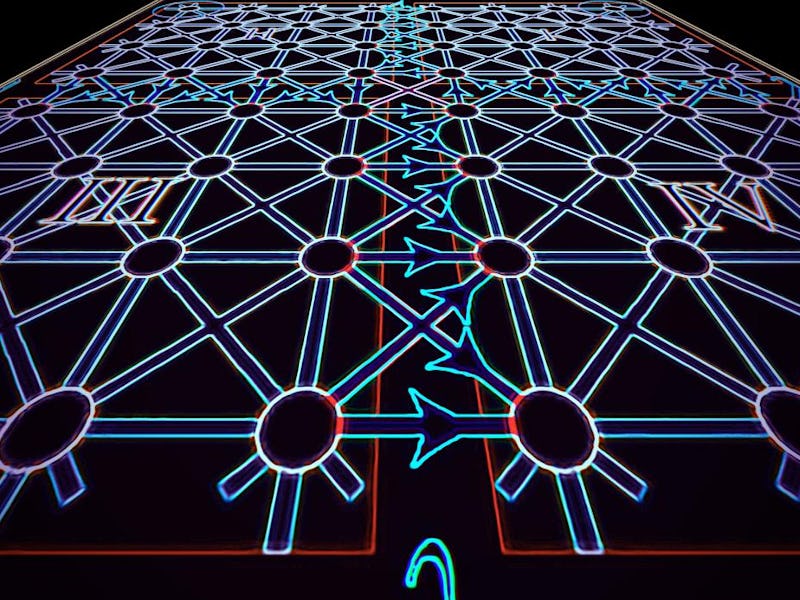
A model of the twisted bulk-boundary-correspondence conditions from the second Science paper.
However, being based in mathematics, the results of this first study were intriguing but still highly theoretical. It took a second paper, complete with a life-sized, 3D printed crystalline structure, to bring the results to life. In the second paper, also published Thursday in the journal Science, researchers explored how twisted bulk-boundary-correspondence could be physically demonstrated. Being in the world of large, Newtonian physics and no longer the small, quantum world of electrons, the research team used sound waves to represent minuscule electron wave functions. Through experiments, the team was able to demonstrate how twisted bulk-boundary-correspondence could occur on the surface as well as how manipulating the sound waves could change the flow of "electrons" on the surface as well.
The lead author of the second paper and physicist at ETH Zurich, Sebastian Huber, said in a statement that these unusual theoretical and experimental results point toward a new overarching understanding of these kinds of materials.
"This was a very left-field idea and realization," Huber said. "We can now show that virtually all topological states that have been realized in our artificial systems are fragile, and not stable as was thought in the past. This work provides that confirmation, but much more, it introduces a new overarching principle."
And when it comes to further research of this property, Bernevig tells Inverse that there's much left to be explored.
"Many things [are left to explore]," says Bernevig. "[W]e know absolutely nothing abt how these states respond to other stimuli: disorder, electric and magnetic fields etc. we know nothing about what happens to these states in the presence of strong interactions. Moreover, the physics breakthrough of last year, a material called twisted bilayer graphene, is theoretically thought to exhibit fragile topology in the bands of interest. Understanding how the topology adds to the remarkable properties of such a material will be crucial to figuring out its puzzles"
Abstract for paper 1: A topological insulator reveals its nontrivial bulk through the presence of gapless edge states: This is called the bulk-boundary correspondence. However, the recent discovery of “fragile” topological states with no gapless edges casts doubt on this concept. We propose a generalization of the bulk-boundary correspondence: a transformation under which the gap between the fragile phase and other bands must close. We derive specific twisted boundary conditions (TBCs) that can detect all the two-dimensional eigenvalue fragile phases. We develop the concept of real-space invariants, local good quantum numbers in real space, which fully characterize these phases and determine the number of gap closings under the TBCs. Realizations of the TBCs in metamaterials are proposed, thereby providing a route to their experimental verification.
Abstract for paper 2: Symmetries crucially underlie the classification of topological phases of matter. Most materials, both natural as well as architectured, possess crystalline symmetries. Recent theoretical works unveiled that these crystalline symmetries can stabilize fragile Bloch bands that challenge our very notion of topology: Although answering to the most basic definition of topology, one can trivialize these bands through the addition of trivial Bloch bands. Here, we fully characterize the symmetry properties of the response of an acoustic metamaterial to establish the fragile nature of the low-lying Bloch bands. Additionally, we present a spectral signature in the form of spectral flow under twisted boundary conditions
This article has been updated to include original comment from the researcher.